ISI MMath Solutions and Discussion 2018 : PMB
ISI MMath PMB 2018 Subjective Questions along with hints and solutions and discussions
Note: You must try the problems on your own before looking at the solutions. I have made the solutions so that you don’t have to join any paid program to get the solutions. After you have failed several times in your attempt to solve a question even with the hints provided, then only look at the solution to that problem. If you cannot solve a single question on your own then this exam is not for you. And if you like my work then please do share this among your friends and do comment below.
To view ISI MMATH 2020 solutions, hints, and discussions: Click Here
To view ISI MMATH 2019 solutions, hints, and discussions: Click Here
To view ISI MMATH 2017 solutions, hints, and discussions: Click Here
To view ISI MMATH 2016 solutions, hints, and discussions: Click Here
To view previous year’s question papers: Click Here
Problem 1.
Find the values of ![]() for which the improper integral
for which the improper integral
![]()
converges.
Topic: Real Analysis
Difficulty level: Medium
Indian Statistical Institute, ISI MMath 2018 Problem 1 Hints along with Solution & Discussion
Hint 1
The integral is defined as the limit of proper intergals
![]()
![]()
Hint 2
Using the relation ![]() we find
we find ![]() such that for all
such that for all ![]() we have
we have ![]()
Hint 3
Then for all ![]() we have a two-sided estimate
we have a two-sided estimate
![]()
and
![]()
Full Solution
The integral is defined as the limit of proper intergals
![]()
![]()
Using the relation ![]() we find
we find ![]() such that for all
such that for all ![]() we have
we have ![]() Then for all
Then for all ![]() we have a two-sided estimate
we have a two-sided estimate
![]()
and
![]()
So, existence of the limit ![]() is equivalent to finiteness of the integral
is equivalent to finiteness of the integral ![]() So,
So,
![]()
Let ![]() We have an estimate
We have an estimate
![Rendered by QuickLaTeX.com \[ \left|\int^C_1 \frac{\sin x}{x^a}dx-\int^{C'}_1 \frac{\sin x}{x^a}dx\right|=\left| \int^{C'}_C\frac{\sin x}{x^a}dx\right|= \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-adf5be16bf12792b839c90fe0d3c5db2_l3.png)
integrate by parts with ![]() so that
so that ![]()
![]()
![Rendered by QuickLaTeX.com \[ =\left| \frac{\cos C}{C^a}-\frac{\cos C'}{(C')^a}-a\int^{C'}_C\frac{\cos x}{x^{a+1}}dx\right|\leq \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-feb40bfc7b50ad673da39981d2396e51_l3.png)
![]()
So, the limit ![]() exists for any
exists for any ![]()
The improper integral ![]() converges if and only if
converges if and only if ![]()
Problem 2.
Let ![]() be a real-valued continuous function which is differentiable on
be a real-valued continuous function which is differentiable on ![]() and satisfies
and satisfies ![]() Suppose there exists a
Suppose there exists a
constant ![]() such that
such that
![]()
Show that ![]() for all
for all ![]() .
.
Topic: Real Analysis
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2018 Problem 2 Hints along with Solution & Discussion
Hint 1
Take ![]() and think of a way to bound
and think of a way to bound ![]() .
.
Hint 2
For any ![]() we have
we have
![]()
Hint 3
Try to show that ![]() .
.
Full Solution
Denote ![]() For any
For any ![]() we have
we have
![]()
Since the estimate holds for any ![]() we take maximum in
we take maximum in ![]() and get
and get
![]()
The assumption ![]() implies
implies ![]() i.e.
i.e. ![]() and
and ![]() for all
for all ![]() .
.
Problem 3.
Let ![]() be an abelian group of order
be an abelian group of order ![]()
(a) If ![]() is a function, then prove that for all
is a function, then prove that for all ![]() ,
,
![]()
(b) Let ![]() be the multiplicative group of non-zero complex numbers and suppose
be the multiplicative group of non-zero complex numbers and suppose ![]() is a homomorphism. Prove that
is a homomorphism. Prove that
![]()
(c) If ![]() is any homomorphism, then prove that
is any homomorphism, then prove that
![]()
Topic: Group Theory
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2018 Problem 3 Hints along with Solution & Discussion
Hint 1
(a) For any ![]() the mapping
the mapping ![]() is a bijection of
is a bijection of ![]() .
.
Hint 2
(b) For any ![]() we have
we have
![]()
Hint 3
(c) Identity
![]()
implies that ![]() is also a homomorphism.
is also a homomorphism.
Full Solution
(a) For any ![]() the mapping
the mapping ![]() is a bijection of
is a bijection of ![]() When
When ![]() ranges through
ranges through ![]() the product
the product ![]() also ranges through
also ranges through ![]()
![]()
(b) For any ![]() we have
we have
![]()
Taking the sum in ![]() we get
we get
![]()
So,
![Rendered by QuickLaTeX.com \[ \left(\sum_{g\in G}f(g)\right)^2=n\sum_{g\in G}f(g), \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-ec9d3a4881b1b847dc4d35a03c1da992_l3.png)
![Rendered by QuickLaTeX.com \[ \sum_{g\in G}f(g)\left(n-\sum_{g\in G}f(g)\right)=0. \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-ed557b9d2574b3b707bb72a4768cf101_l3.png)
Either ![]() or
or ![]()
(c) Identity
![]()
implies that ![]() is also a homomorphism. By previous part, either
is also a homomorphism. By previous part, either
![]() or
or ![]() For all
For all ![]() we have
we have ![]()
![]() and
and ![]() It follows that
It follows that ![]()
Problem 4.
(a) Is the ideal ![]() in the polynomial ring
in the polynomial ring ![]() a prime ideal? Justify your answer.
a prime ideal? Justify your answer.
(b) Is the ideal ![]() in the polynomial ring
in the polynomial ring ![]() a prime ideal? Justify your answer.
a prime ideal? Justify your answer.
Topic: Ring Theory
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2018 Problem 4 Hints along with Solution & Discussion
Hint 1
(a) Try to use the relation
![]()
Hint 2
Assume that ![]() is a prime ideal in
is a prime ideal in ![]() Consider polynomial
Consider polynomial ![]() Then either
Then either ![]() or
or ![]()
Hint 3
(b) Hint continued:
General element of ![]() is of the form
is of the form
![]()
Full Solution
(a) Relations
![]()
imply that ![]() General element of
General element of ![]() is of the form
is of the form ![]() i.e.
i.e.
![]()
If ![]() then
then ![]() which is possible only when
which is possible only when ![]() or
or ![]()
![]() is a prime ideal.
is a prime ideal.
(b) Assume that ![]() is a prime ideal in
is a prime ideal in ![]() Consider polynomial
Consider polynomial ![]() Then either
Then either ![]() or
or ![]() General element of
General element of ![]() is of the form
is of the form
![]()
In particular, an element of ![]() vanished when
vanished when ![]() So,
So, ![]() It follows that
It follows that ![]() and
and
![]()
Then, ![]() and
and
![]()
which is impossible in ![]()
![]() is not a prime ideal.
is not a prime ideal.
Problem 5.
Let ![]() and
and ![]() be an
be an ![]() matrix with real entries. Let
matrix with real entries. Let ![]() denote the adjoint of
denote the adjoint of ![]() that is, the
that is, the ![]() -th entry of
-th entry of ![]() is the
is the
![]() -th cofactor of
-th cofactor of ![]() Show that the rank of
Show that the rank of ![]() is
is ![]() ,
, ![]() or
or ![]()
Topic: Linear Algebra
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2018 Problem 5 Hints along with Solution & Discussion
Hint 1
If ![]() then the inverse of
then the inverse of ![]() is given by
is given by ![]() In this case the rank of
In this case the rank of ![]() is
is ![]()
Hint 2
Assume that the rank of ![]() is
is ![]() Then each
Then each ![]() minor of
minor of ![]() is zero, and all elements of
is zero, and all elements of ![]() are equal to zero. In this case the rank of
are equal to zero. In this case the rank of ![]() is equal to zero.
is equal to zero.
Hint 3
Assume that the rank of ![]() is equal to
is equal to ![]() Then there exists a nonzero
Then there exists a nonzero ![]() minor of
minor of ![]() so the rank of
so the rank of ![]() is
is ![]() Denote by
Denote by ![]() the
the ![]() th cofactor of
th cofactor of ![]() . Then
. Then ![]() Consider the product
Consider the product
![]() Its
Its ![]() th entry is
th entry is
![Rendered by QuickLaTeX.com \[ \sum^n_{k=1}a_{ik}( \mbox{Adj} A)_{kj}=\sum^n_{k=1}a_{ik}a^{(jk)}=0 \eqno(1) \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-9304efdbece209b52d5e6c6958da7f2e_l3.png)
Full Solution
If ![]() then the inverse of
then the inverse of ![]() is given by
is given by ![]() In this case the rank of
In this case the rank of ![]() is
is ![]()
Assume that the rank of ![]() is
is ![]() Then each
Then each ![]() minor of
minor of ![]() is zero, and all elements of
is zero, and all elements of ![]() are equal to zero. In this case the rank of
are equal to zero. In this case the rank of ![]() is equal to zero.
is equal to zero.
Assume that the rank of ![]() is equal to
is equal to ![]() Then there exists a nonzero
Then there exists a nonzero ![]() minor of
minor of ![]() so the rank of
so the rank of ![]() is
is ![]() Denote by
Denote by ![]() the
the ![]() th cofactor of
th cofactor of ![]() . Then
. Then ![]() Consider the product
Consider the product
![]() Its
Its ![]() th entry is
th entry is
![Rendered by QuickLaTeX.com \[ \sum^n_{k=1}a_{ik}( \mbox{Adj} A)_{kj}=\sum^n_{k=1}a_{ik}a^{(jk)}=0 \eqno(1) \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-9304efdbece209b52d5e6c6958da7f2e_l3.png)
Indeed, if ![]() then the sum in (1) is the determinant of
then the sum in (1) is the determinant of ![]() If
If ![]() then the sum in (1) is the determinant of the matrix
then the sum in (1) is the determinant of the matrix ![]() after the
after the ![]() th row is replaced with the
th row is replaced with the ![]() th row. Such determinant is zero as the matrix has two identical rows. We see that
th row. Such determinant is zero as the matrix has two identical rows. We see that ![]() That is, each column of
That is, each column of ![]() is in the kernel of
is in the kernel of ![]() But the rank of
But the rank of ![]() is
is ![]() , hence the dimension of the kernel of
, hence the dimension of the kernel of ![]() is
is ![]() and the dimension of the column range of
and the dimension of the column range of ![]() is
is ![]() This proves that the rank of
This proves that the rank of ![]() is equal to 1.
is equal to 1.
Problem 6.
Suppose an urn contains a red ball and a blue ball. A ball is drawn at random and a ball of the same colour is added to the urn along with
the one that was drawn. This process is repeated indefinitely.
Let ![]() denote the random variable that takes the value
denote the random variable that takes the value ![]() if the first
if the first ![]() draws yield red balls and the
draws yield red balls and the ![]() -th draw yields a blue ball.
-th draw yields a blue ball.
(a) If ![]() , find
, find ![]() .
.
(b) Show that the probability of a blue ball being chosen eventually is ![]()
(c) Find ![]()
Topic: Probability
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2018 Problem 6 Hints along with Solution & Discussion
Hint 1
(a) We use induction on ![]() Let
Let ![]() The event
The event ![]() means that the first draw yielded a red ball,
means that the first draw yielded a red ball,
![]()
Hint 2
(b) If the blue ball is never chosen, then ![]() for all
for all ![]() Denote by
Denote by ![]() the event when the blue ball is never chosen. Then
the event when the blue ball is never chosen. Then
![]()
So, ![]()
Hint 3
(c) For each ![]() we have
we have
![]()
Full Solution
(a) We use induction on ![]() Let
Let ![]() The event
The event ![]() means that the first draw yielded a red ball,
means that the first draw yielded a red ball,
![]()
Assume that event ![]() happens. Then first
happens. Then first ![]() draws yielded red balls, and before the
draws yielded red balls, and before the ![]() st draw there are
st draw there are ![]() red balls and
red balls and ![]() blue ball in the urn. The probability to draw a red ball is
blue ball in the urn. The probability to draw a red ball is ![]() So,
So,
![]()
If ![]() then
then ![]() For
For ![]() the equality
the equality ![]() is true. So, for all
is true. So, for all ![]()
![]()
(b) If the blue ball is never chosen, then ![]() for all
for all ![]() Denote by
Denote by ![]() the event when the blue ball is never chosen. Then
the event when the blue ball is never chosen. Then
![]()
So, ![]()
(c) For each ![]() we have
we have
![]()
Then
![Rendered by QuickLaTeX.com \[ E[X]=\sum^\infty_{n=1}nP(X=n)=\sum^\infty_{n=1}\frac{1}{n+1}=\infty. \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-c33b4e394ba04eedcd25fdecab4c368c_l3.png)
Problem 7.
A real number ![]() is said to be a limit point of a set
is said to be a limit point of a set ![]() if every
if every
neighbourhood of ![]() contains a point of
contains a point of ![]() other than
other than ![]() Consider the
Consider the
set
![]()
(a) Show that ![]() contains infinitely many limit points of
contains infinitely many limit points of ![]()
(b) Show that ![]() is a compact subset of
is a compact subset of ![]()
(c) Find all limit points of ![]()
Topic: Real Analysis
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2018 Problem 7 Hints along with Solution & Discussion
Hint 1
(a) Observe that ![]() Let
Let ![]() and
and ![]() Then
Then
![]()
Hint 2
(b) The set ![]() is bounded:
is bounded: ![]() It remains to prove that
It remains to prove that ![]() is closed.
is closed.
Hint 3
(c) Considerations of previous two parts shows that the limit points of ![]() are
are ![]()
Full Solution
(a) Observe that ![]() Let
Let ![]() and
and ![]() Then
Then
![]()
Every neighborhood of ![]() contains a point from
contains a point from ![]() distinct from
distinct from ![]() Each point
Each point ![]() is a limit point of the set
is a limit point of the set ![]() that belongs to the set
that belongs to the set ![]()
If ![]() then
then ![]() is a point of
is a point of ![]() in the
in the ![]() neighborhood of
neighborhood of ![]() .
. ![]() is a limit point of
is a limit point of ![]() We’ve proved that points
We’ve proved that points
![]()
are limit points of ![]() .
.
(b)
The set ![]() is bounded:
is bounded: ![]() It remains to prove that
It remains to prove that ![]() is closed. Suppose that
is closed. Suppose that ![]() Every neighborhood of
Every neighborhood of ![]() contains a point
contains a point ![]() . But
. But ![]() and
and ![]() Then
Then ![]() is a limit point of
is a limit point of ![]() Since we assume
Since we assume ![]() it follows that
it follows that ![]() For every
For every ![]() find a point
find a point ![]() such that
such that
![]()
If both sequences ![]() and
and ![]() are bounded, then there exist values
are bounded, then there exist values ![]() such that
such that ![]() and
and ![]() for infinitely many values of
for infinitely many values of ![]() But then
But then ![]() which is not the case. Hence we can assume that
which is not the case. Hence we can assume that ![]() is unbounded. Let
is unbounded. Let ![]() be a subsequence such that
be a subsequence such that ![]() If the sequence
If the sequence ![]() is bounded, there exists value
is bounded, there exists value ![]() such that
such that ![]() for infinitely many values of
for infinitely many values of ![]() But then
But then
![]() If the sequence
If the sequence ![]() is unbounded, we can pass to another subsequence
is unbounded, we can pass to another subsequence ![]() that converges to
that converges to ![]() Then we have
Then we have ![]() In any case
In any case ![]() and
and ![]() is compact.
is compact.
(c) Considerations of previous two parts shows that the limit points of ![]() are
are ![]()
Problem 8.
(a) Let ![]() be a sequence of continuous functions on
be a sequence of continuous functions on ![]() such
such
that ![]() converges uniformly on
converges uniformly on ![]() Show that
Show that ![]()
converges.
(b) Find the set ![]() of all points
of all points ![]() such that the series
such that the series
![Rendered by QuickLaTeX.com \[ \sum^\infty_{n=1}e^{-nx}\cos(nx) \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-22f4e6642fa987302c4cb3502b66fa3c_l3.png)
converges. Does this series converge uniformly on ![]() ? Justify your answer.
? Justify your answer.
Topic: Real Analysis
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2018 Problem 8 Hints along with Solution & Discussion
Hint 1
(a) Denote ![]() The sequence
The sequence ![]() converges uniformly on
converges uniformly on ![]() there exists function
there exists function ![]() on
on ![]() such that
such that
![]()
Hint 2
(a) Hint Continued: Verify that ![]() exists. Let
exists. Let ![]() Find
Find ![]() such that
such that
![]()
Hint 3
(b) The series converges for all ![]() Indeed,
Indeed,
![Rendered by QuickLaTeX.com \[ \sum^\infty_{n=1}|e^{-nx}\cos(nx)|\leq \sum^\infty_{n=1}e^{-nx}=\frac{e^{-x}}{1-e^{-x}}<\infty. \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-f20a91d321114a603b6d38c68e7740fc_l3.png)
Full Solution
(a) Denote ![]() The sequence
The sequence ![]() converges uniformly on
converges uniformly on ![]() there exists function
there exists function ![]() on
on ![]() such that
such that
![]()
We verify that ![]() exists. Let
exists. Let ![]() Find
Find ![]() such that
such that
![]()
Using continuity of ![]() find
find ![]() such that for all
such that for all ![]()
![]()
Then for all ![]() we have
we have
![]()
Since ![]() is arbitrary, this shows that
is arbitrary, this shows that ![]() exists. We denote the limit by
exists. We denote the limit by ![]()
Now, let ![]() Find
Find ![]() such that for all
such that for all ![]() and all
and all ![]() we have
we have
![]()
Passing to the limit as ![]() we get
we get
![]()
Since ![]() is arbitrary, this shows that
is arbitrary, this shows that
![Rendered by QuickLaTeX.com \[ s(0)=\lim_{m\to\infty}s_m(0)=\lim_{m\to\infty}\sum^m_{n=1}f_n(0), \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-4f509632b071b884e13ce72418b00223_l3.png)
i.e. ![]()
converges and has the sum ![]() .
.
(b) The series converges for all ![]() Indeed,
Indeed,
![Rendered by QuickLaTeX.com \[ \sum^\infty_{n=1}|e^{-nx}\cos(nx)|\leq \sum^\infty_{n=1}e^{-nx}=\frac{e^{-x}}{1-e^{-x}}<\infty. \]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-f20a91d321114a603b6d38c68e7740fc_l3.png)
For ![]() the series diverges, as it is the series
the series diverges, as it is the series ![]() So,
So, ![]() If the series converges uniformly, then it should also converge at
If the series converges uniformly, then it should also converge at ![]() So, the series
So, the series ![]() does not converge uniformly on
does not converge uniformly on ![]()

Sir,my daughter wants to prepare for m.math entrance examination..can you please help her with your classes and practice sets??
Hello, Yes I can help her out with my tutoring. There is a batch for M.Math where I teach every Sunday starting from 5 pm onwards. For further detail, you can contact me at +91-8247590347 or email me at admin@fractionshub.com.
For Problem 2, Can I solve this like this?
Let![Rendered by QuickLaTeX.com x \in [0,1]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-70927c43322f0011d696d05cd2c78c56_l3.png) then by using MVT there exists
then by using MVT there exists  such that
such that  i.e
i.e  . Similary there exists
. Similary there exists  such that
such that  and applying modulus we get
and applying modulus we get  So with the above inequalities we have
So with the above inequalities we have  . Proceeding this way we have
. Proceeding this way we have 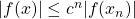 but since
but since  is continuous on
is continuous on ![Rendered by QuickLaTeX.com [0,1]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-e4206fd46f63fb93a794d3d340fa3b28_l3.png) so is
so is  so it attains the maximum say
so it attains the maximum say 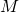 then the inequality becomes
then the inequality becomes 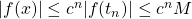 and we know that
and we know that  . so for any
. so for any 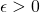 there exists
there exists  such that for all
such that for all  we have
we have  In particular
In particular 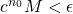 hence
hence 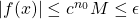 this implies
this implies 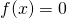 and since
and since  was arbitrary we have
was arbitrary we have ![Rendered by QuickLaTeX.com f(x) = 0 \forall x \in [0,1]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-8cabeaa9614f8d4adaa42e2531dee505_l3.png)
For Problem 2, Can I solve this like this?
Let![Rendered by QuickLaTeX.com x \in [0,1]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-70927c43322f0011d696d05cd2c78c56_l3.png) then by using MVT there exists
then by using MVT there exists  such that
such that  i.e
i.e  . Similary there exists
. Similary there exists  such that
such that  and applying modulus we get
and applying modulus we get  So with the above inequalities we have
So with the above inequalities we have  . Proceeding this way we have
. Proceeding this way we have 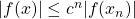 but since
but since  is continuous on
is continuous on ![Rendered by QuickLaTeX.com [0,1]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-e4206fd46f63fb93a794d3d340fa3b28_l3.png) so is
so is  so it attains the maximum say
so it attains the maximum say 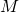 then the inequality becomes
then the inequality becomes 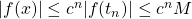 and we know that
and we know that  . so for any
. so for any 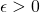 there exists
there exists  such that for all
such that for all  we have
we have  In particular
In particular 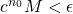 hence
hence 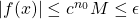 this implies
this implies 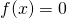 and since
and since  was arbitrary we have
was arbitrary we have ![Rendered by QuickLaTeX.com f(x) = 0 \forall x \in [0,1]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-8cabeaa9614f8d4adaa42e2531dee505_l3.png)