ISI MMath Solutions and Discussion 2019 : PMB
ISI MMath PMB 2019 Subjective Questions along with hints and solutions and discussions
To view ISI MMATH 2020 solutions, hints, and discussions: Click Here
To view ISI MMATH 2018 solutions, hints, and discussions: Click Here
To view ISI MMATH 2017 solutions, hints, and discussions: Click Here
To view ISI MMATH 2016 solutions, hints, and discussions: Click Here
To view previous year’s question papers: Click Here
Problem 1.
Let ![]() be a continuous function satisfying
be a continuous function satisfying
![]()
Show that ![]() is a bounded function on
is a bounded function on ![]() and attains a maximum or a minimum. Give an example to show that it attains a maximum but not a minimum.
and attains a maximum or a minimum. Give an example to show that it attains a maximum but not a minimum.
Topic: Real Analysis
Difficulty level: Medium
Indian Statistical Institute, ISI MMath 2019 Problem 1 Hints along with Solution & Discussion
Hint 1
Assume that ![]() is non-negative, i.e.
is non-negative, i.e. ![]() for all
for all ![]() If
If ![]() for all
for all ![]() then obviously
then obviously ![]() is bounded and attains its maximal (and minimal) value at each point. Let
is bounded and attains its maximal (and minimal) value at each point. Let ![]() for some
for some ![]() By assumption, there exists
By assumption, there exists ![]() such that for all
such that for all ![]() and all
and all ![]()
![]() Let
Let ![]() be the restriction of
be the restriction of ![]() to
to ![]()
![]() is a continuous function on a compact segment.
is a continuous function on a compact segment.
Hint 2
Let ![]() be a continuous function satisfying
be a continuous function satisfying
![]()
The function ![]() is a non-negative continuous function satisfying
is a non-negative continuous function satisfying
![]()
Hint 3
For the Example part, consider the function ![]()
Full Solution
Step 1:
Assume that ![]() is non-negative, i.e.
is non-negative, i.e. ![]() for all
for all ![]() If
If ![]() for all
for all ![]() then obviously
then obviously ![]() is bounded and attains its maximal (and minimal) value at each point. Let
is bounded and attains its maximal (and minimal) value at each point. Let ![]() for some
for some ![]() By assumption, there exists
By assumption, there exists ![]() such that for all
such that for all ![]() and all
and all ![]()
![]() Let
Let ![]() be the restriction of
be the restriction of ![]() to
to ![]()
![]() is a continuous function on a compact segment. Hence,
is a continuous function on a compact segment. Hence, ![]() is bounded and attains its maximal value on
is bounded and attains its maximal value on ![]() i.e. there exists
i.e. there exists ![]() such that
such that ![]() We check that the function
We check that the function ![]() attains its maximal value at
attains its maximal value at ![]() Let
Let ![]() Then
Then
![]()
Let ![]() or
or ![]() Then
Then ![]() and
and
![]()
So, for each ![]()
![]()
![]() is bounded and attains its maximal value.
is bounded and attains its maximal value.
Step 2:
Let ![]() be a continuous function satisfying
be a continuous function satisfying
![]()
The function ![]() is a non-negative continuous function satisfying
is a non-negative continuous function satisfying
![]()
By step 1, ![]() is bounded and attains its maximal value. It means that there exists
is bounded and attains its maximal value. It means that there exists ![]() such that for all
such that for all ![]()
![]()
So, for all ![]()
![]()
The function ![]() is bounded. If
is bounded. If ![]() then
then ![]() and
and ![]() attains its maximal value. If
attains its maximal value. If ![]() then
then ![]() and
and ![]() attains its minimal value.
attains its minimal value.
Step 3:
Consider the function ![]() Then
Then ![]() is a continuous non-negative function,
is a continuous non-negative function,
![]()
In particular, ![]() Further,
Further,
![]()
Function ![]() attains its maximal value at
attains its maximal value at ![]() However, for all
However, for all ![]()
![]() and the function
and the function ![]() does not attain its minimal value.
does not attain its minimal value.
Problem 2.
Let ![]() be a continuous function such that
be a continuous function such that ![]() Show
Show
that
![]()
Topic: Real Analysis
Difficulty Level: Easy
Indian Statistical Institute, ISI MMath 2019 Problem 2 Hints along with Solution & Discussion
Hint 1
Function ![]() is bounded, as it is a continuous function on a compact
is bounded, as it is a continuous function on a compact ![]() Let
Let ![]() Fix
Fix ![]() By assumption, there exists
By assumption, there exists ![]() such that for all
such that for all ![]()
![]() Let
Let ![]() be such that
be such that ![]()
Hint 2
Let ![]() and
and ![]() If
If ![]() then
then
![]()
Hint 3
If ![]() then
then
![]()
Full Solution
Function ![]() is bounded, as it is a continuous function on a compact
is bounded, as it is a continuous function on a compact ![]() Let
Let ![]() Fix
Fix ![]() By assumption, there exists
By assumption, there exists ![]() such that for all
such that for all ![]()
![]() Let
Let ![]() be such that
be such that ![]()
Let ![]() and
and ![]() If
If ![]() then
then
![]()
If ![]() then
then
![]()
In any case,
![]() and
and
![]()
Since ![]() is arbitrary, this proves the needed convergence.
is arbitrary, this proves the needed convergence.
Problem 3.
Let ![]() be a twice continuously differentiable function and
be a twice continuously differentiable function and
suppose ![]() If
If ![]() for all
for all ![]() then prove that
then prove that
![]() for all
for all ![]()
Topic: Real Analysis
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2019 Problem 3 Hints along with Solution & Discussion
Hint 1
For every ![]() we have by the Newton-Leibniz formula
we have by the Newton-Leibniz formula
![]()
Hint 2
Applying the Newton-Leibniz rule again we get
![]()
Hint 3
In particular, for each ![]() we have
we have
![]()
Full Solution
For every ![]() we have by the Newton-Leibniz formula
we have by the Newton-Leibniz formula
![]()
Applying the Newton-Leibniz rule again we get
![]()
In particular, for each ![]() we have
we have
![]()
Problem 4.
Suppose ![]() is a function defined by
is a function defined by
![Rendered by QuickLaTeX.com \[f(x,y)=\begin{cases} \frac{x^2y^3}{x^4+y^6}, \mbox{ if } x\ne 0, y\in\mathbb{R}, \\ 0, \mbox{ if } x=0, y\in\mathbb{R}. \end{cases}\]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-74d6446cc95ee1e93b85e7b5d7ca047f_l3.png)
(a). Find all ![]() such that
such that ![]() has a nonzero directional derivative at
has a nonzero directional derivative at ![]() with respect to the direction
with respect to the direction ![]()
(b). Is ![]() continuous at
continuous at ![]() Justify your answer.
Justify your answer.
Topic: Multivariable Calculus
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2019 Problem 4 Hints along with Solution & Discussion
Hint 1
Part a.
Let ![]()
![]() Then the directional derivative at
Then the directional derivative at ![]() with respect to the direction
with respect to the direction ![]() is
is
![]()
Hint 2
Let ![]() Then the directional derivative at
Then the directional derivative at ![]() with respect to the direction
with respect to the direction ![]() is
is
![]()
![]()
Hint 3
Part b.
If ![]() is continuous at
is continuous at ![]() , then for any sequence
, then for any sequence ![]() we have
we have ![]() Let
Let ![]()
![]()
Full Solution
Part a.
Let ![]()
![]() Then the directional derivative at
Then the directional derivative at ![]() with respect to the direction
with respect to the direction ![]() is
is
![]()
Let ![]() Then the directional derivative at
Then the directional derivative at ![]() with respect to the direction
with respect to the direction ![]() is
is
![]()
![]()
![]() has a nonzero directional derivative at
has a nonzero directional derivative at ![]() with respect to the direction
with respect to the direction ![]() if and only if
if and only if ![]() and
and ![]()
Part b.
If ![]() is continuous at
is continuous at ![]() , then for any sequence
, then for any sequence ![]() we have
we have ![]() Let
Let ![]()
![]() Then
Then
![]()
So, ![]() is not continuous at
is not continuous at ![]()
Problem 5.
Let ![]() be a subset of a compact metric space
be a subset of a compact metric space ![]() Assume that for
Assume that for
every continuous function ![]() , the restriction of
, the restriction of ![]() to
to ![]() attains
attains
a maximum on ![]() Prove that
Prove that ![]() is compact.
is compact.
Topic: Metric Space
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2019 Problem 5 Hints along with Solution & Discussion
Hint 1
A subset of a compact metric space is compact if and only if it is a closed subset. So, it is enough to check that ![]() is a closed subset of
is a closed subset of ![]()
Hint 2
Let ![]() Consider function
Consider function ![]()
![]() is a continuous function on
is a continuous function on ![]()
Hint 3
Indeed, by the triangle inequality,
![]()
So, ![]() attains a maximum on
attains a maximum on ![]() . Since
. Since ![]() it follows that there exists a point
it follows that there exists a point ![]() such that
such that ![]()
Full Solution
A subset of a compact metric space is compact if and only if it is a closed subset. So, it is enough to check that ![]() is a closed subset of
is a closed subset of ![]() Let
Let ![]() Consider function
Consider function ![]()
![]() is a continuous function on
is a continuous function on ![]() Indeed, by the triangle inequality,
Indeed, by the triangle inequality,
![]()
So, ![]() attains a maximum on
attains a maximum on ![]() . Since
. Since ![]() it follows that there exists a point
it follows that there exists a point ![]() such that
such that ![]() Then
Then ![]() and
and ![]() So,
So, ![]() and
and ![]() is closed.
is closed.
Problem 6.
Let ![]() be a non-abelian group of order
be a non-abelian group of order ![]() , where
, where ![]() are primes.
are primes.
(a). How many elements of ![]() have order
have order ![]()
(b). How many elements of ![]() have order
have order ![]()
Topic: Group Theory
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2019 Problem 6 Hints along with Solution & Discussion
Hint 1
The order of an element divides the order of a group. So each element of ![]() is of order
is of order ![]() or
or ![]() Since
Since ![]() is non-abelian, it has no elements of order
is non-abelian, it has no elements of order ![]() By Cauchy’s theorem, there exists an element
By Cauchy’s theorem, there exists an element ![]() of order
of order ![]() Then each of the elements
Then each of the elements
![]()
is of order ![]() .
.
Hint 2
Assume that ![]() is of order
is of order ![]() and
and ![]()
![]() Consider subgroups
Consider subgroups
![]()
and
![]()
Hint 3
Then ![]() is a subgroup of
is a subgroup of ![]() strictly smaller than
strictly smaller than ![]() (as
(as ![]() ). The order of
). The order of ![]() is prime, hence
is prime, hence ![]() Consider cosets
Consider cosets
![]()
The size of each coset is ![]() and there are
and there are ![]() cosets.
cosets. ![]() hence two of different cosets intersect:
hence two of different cosets intersect:
![]()
Full Solution
The order of an element divides the order of a group. So each element of ![]() is of order
is of order ![]() or
or ![]() Since
Since ![]() is non-abelian, it has no elements of order
is non-abelian, it has no elements of order ![]() By Cauchy’s theorem, there exists an element
By Cauchy’s theorem, there exists an element ![]() of order
of order ![]() Then each of the elements
Then each of the elements
![]()
is of order ![]() .
.
Assume that ![]() is of order
is of order ![]() and
and ![]()
![]() Consider subgroups
Consider subgroups
![]()
and
![]()
Then ![]() is a subgroup of
is a subgroup of ![]() strictly smaller than
strictly smaller than ![]() (as
(as ![]() ). The order of
). The order of ![]() is prime, hence
is prime, hence ![]() Consider cosets
Consider cosets
![]()
The size of each coset is ![]() and there are
and there are ![]() cosets.
cosets. ![]() hence two of different cosets intersect:
hence two of different cosets intersect:
![]()
We deduce that there exists ![]() such that
such that ![]() But then
But then ![]()
![]() which contradicts the fact that
which contradicts the fact that ![]() is of order
is of order ![]()
Conclusion: all elements of order ![]() are among
are among ![]()
(a). There are ![]() elements of order
elements of order ![]()
(b). There are ![]() elements of order
elements of order ![]()
Problem 7.
Prove or disprove the following statement: The ring ![]() is isomorphic to a product of fields.
is isomorphic to a product of fields.
Topic: Field Theory
Difficulty Level: Hard
Indian Statistical Institute, ISI MMath 2019 Problem 7 Hints along with Solution & Discussion
Hint 1
We prove that ![]() is isomorphic to a product of three fields. Consider the factorization
is isomorphic to a product of three fields. Consider the factorization
![]()
Hint 2
Consider ideals ![]()
![]()
![]()
Hint 3
Relations
![]()
![]()
imply that ideals ![]() are pairwise coprime.
are pairwise coprime.
Now try to apply the Chinese remainder theorem.
Full Solution
We prove that ![]() is isomorphic to a product of three fields. Consider the factorization
is isomorphic to a product of three fields. Consider the factorization
![]()
Consider ideals ![]()
![]()
![]() Relations
Relations
![]()
![]()
imply that ideals ![]() are pairwise coprime. By the Chinese remainder theorem,
are pairwise coprime. By the Chinese remainder theorem,
![]()
Each of the multiples in the right-hand side is a field, as it is a quotient of ![]() by an irreducible polynomial. In fact,
by an irreducible polynomial. In fact,
![]()
The ring ![]() is isomorphic to a product of three fields
is isomorphic to a product of three fields ![]()
Problem 8.
Let ![]() be a symmetric matrix with real entries such that
be a symmetric matrix with real entries such that ![]() for some
for some ![]() Show that
Show that ![]()
Topic: Linear Algebra
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2019 Problem 8 Hints along with Solution & Discussion
Hint 1
A symmetric real matrix is diagonalizable
Hint 2
there exists an invertible matrix ![]() and a diagonal matrix
and a diagonal matrix ![]() such that
such that
![Rendered by QuickLaTeX.com \[M=CDC^{-1}, D=\begin{pmatrix} \lambda_1 & 0 & 0 & \ldots & 0 \\ 0& \lambda_2 & 0 & \ldots & 0 \\ 0 & 0 & \lambda_3 & \ldots & 0 \\ \ldots & \ldots & \ldots & \ldots & \ldots \\ 0 & 0 & 0 & \ldots & \lambda_n \end{pmatrix}\]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-8f04aa2ed2485da77cb5ca5d223455fa_l3.png)
Hint 3
Then
![]()
Full Solution
A symmetric real matrix is diagonalizable: there exists an invertible matrix ![]() and a diagonal matrix
and a diagonal matrix ![]() such that
such that
![Rendered by QuickLaTeX.com \[M=CDC^{-1}, D=\begin{pmatrix} \lambda_1 & 0 & 0 & \ldots & 0 \\ 0& \lambda_2 & 0 & \ldots & 0 \\ 0 & 0 & \lambda_3 & \ldots & 0 \\ \ldots & \ldots & \ldots & \ldots & \ldots \\ 0 & 0 & 0 & \ldots & \lambda_n \end{pmatrix}\]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-8f04aa2ed2485da77cb5ca5d223455fa_l3.png)
Then
![]()
So,
![Rendered by QuickLaTeX.com \[D^k=\begin{pmatrix} \lambda^k_1 & 0 & 0 & \ldots & 0 \\ 0& \lambda^k_2 & 0 & \ldots & 0 \\ 0 & 0 & \lambda^k_3 & \ldots & 0 \\ \ldots & \ldots & \ldots & \ldots & \ldots \\ 0 & 0 & 0 & \ldots & \lambda^k_n \\ \end{pmatrix}=0\]](https://fractionshub.com/wp-content/ql-cache/quicklatex.com-c624e55e486d4536bf9e605b3339d466_l3.png)
and ![]()
![]() and
and ![]()
Problem 9.
Suppose ![]() and
and ![]() are two
are two ![]() matrices with real entries such that
matrices with real entries such that
the sum of their ranks is strictly less than ![]() Show that there exists a
Show that there exists a
nonzero column vector ![]() such that
such that ![]()
Topic: Linear Algebra
Difficulty Level: Easy
Indian Statistical Institute, ISI MMath 2019 Problem 9 Hints along with Solution & Discussion
Hint 1
Consider the matrix
![]()
The rank of ![]() is the maximal number of linearly independent rows of
is the maximal number of linearly independent rows of ![]()
Hint 2
Then
![]()
Hint 3
Consequently, columns of ![]() are linearly dependent, and there exists a nonzero vector
are linearly dependent, and there exists a nonzero vector ![]() such that
such that
![]()
It means that ![]()
Full Solution
Consider the matrix
![]()
The rank of ![]() is the maximal number of linearly independent rows of
is the maximal number of linearly independent rows of ![]() Then
Then
![]()
Consequently, columns of ![]() are linearly dependent, and there exists a nonzero vector
are linearly dependent, and there exists a nonzero vector ![]() such that
such that
![]()
It means that ![]()
Problem 10.
Suppose there are ![]() persons in a party. Every pair of persons meet each other with probability
persons in a party. Every pair of persons meet each other with probability ![]() independently of the other pairs.
independently of the other pairs.
Let ![]() be the number of people the
be the number of people the ![]() person meets in the party. For all
person meets in the party. For all ![]() with
with ![]() and for all
and for all ![]()
show that
![]()
![]()
Topic: Probability
Difficulty Level: Medium
Indian Statistical Institute, ISI MMath 2019 Problem 10 Hints along with Solution & Discussion
Hint 1
Introduce two events:
![]()
![]()
Assume the event ![]() happens. Then
happens. Then ![]() meets
meets ![]() and
and ![]() meets
meets ![]() other persons (from
other persons (from ![]() possible persons). There are
possible persons). There are ![]() choices of persons except
choices of persons except ![]() that
that ![]() meets. The probability that
meets. The probability that ![]() meets
meets ![]() persons except
persons except ![]() is
is
![]()
Hint 2
Further, ![]() meets
meets ![]() persons except
persons except ![]() (from
(from ![]() possible persons). There are
possible persons). There are ![]() choices of persons except
choices of persons except ![]() that
that ![]() meets. The probability that
meets. The probability that ![]() meets
meets ![]() persons except
persons except ![]() is
is
![]()
Hint 3
Finally,
![]()
![]()
Full Solution
Introduce two events:
![]()
![]()
Assume the event ![]() happens. Then
happens. Then ![]() meets
meets ![]() and
and ![]() meets
meets ![]() other persons (from
other persons (from ![]() possible persons). There are
possible persons). There are ![]() choices of persons except
choices of persons except ![]() that
that ![]() meets. The probability that
meets. The probability that ![]() meets
meets ![]() persons except
persons except ![]() is
is
![]()
Further, ![]() meets
meets ![]() persons except
persons except ![]() (from
(from ![]() possible persons). There are
possible persons). There are ![]() choices of persons except
choices of persons except ![]() that
that ![]() meets. The probability that
meets. The probability that ![]() meets
meets ![]() persons except
persons except ![]() is
is
![]()
Finally,
![]()
![]()
Assume the event ![]() happens. Then
happens. Then ![]() does not meet
does not meet ![]() and
and ![]() meets
meets ![]() other persons (from
other persons (from ![]() possible persons). There are
possible persons). There are ![]() choices of persons except
choices of persons except ![]() that
that ![]() meets. The probability that
meets. The probability that ![]() meets
meets ![]() persons except
persons except ![]() is
is
![]()
Further, ![]() meets
meets ![]() persons except
persons except ![]() (from
(from ![]() possible persons). There are
possible persons). There are ![]() choices of persons except
choices of persons except ![]() that
that ![]() meets. The probability that
meets. The probability that ![]() meets
meets ![]() persons except
persons except ![]() is
is
![]()
Finally,
![]()
![]()
We deduce that
![]()
![]()

I have tried my best to explain the solutions. If you have some other solutions, please feel free to add them in the comment section. My website is latex enabled so that you can type all the mathematical equations. If you face any problem understanding the solutions, then comment here, and I will get back to you as soon as I can. Also if you find any mathematical error or any typing error then please do comment here.
Sir,
I am from howrah, West Bengal. There is a kind request to you from my side that if u please teach me as a private tutor for preparation for ISI and CMI, I would be highly obliged. I also like your problems and try to figure it out. But I think it would be more clear if you kind see to my above request.
Regards,
Soumyajit Ganguly
Please check your email. I have sent you all the details regarding our classes.
Sir
I am from howrah, West Bengal. There is a kind request to you from my side that if u please teach me as a private tutor for preparation for ISI and CMI, I would be highly obliged. I also like your problems and try to figure it out. But I think it would be more clear if you kind see to my above request.
Please check your email. I have sent you all the details regarding our classes.
Hi!
Can you please conduct few mock tests for ISI M.MATH entrance?
We will try our best to make the mock test course. But currently, we are busy with making some other courses. We will keep you updated.
Sir, it would be really very helpful if you can create a mock test course for this year’s entrance.
In 5th question I got it h(x)<h(x○)=1 but I didn't got how u proved then that C is closed
Showing that C(Bar)=C, that is C contains all it’s limit points. The last line there would be C(bar) but the latex is not taking the symbol bar.
For problem 3, can we use the Taylor’s theorem instead? like for f(x)= f(0) + xf'(0) + (x^2/2)f”(c), for c belongs to (0,x) where for x<0 the case is same because in either way f'(0) = 0 = f(0)
so when we apply |f(x)| =|x^2f"(c)|/2 < 1/2
For problem 3, can we use the Taylor’s theorem instead? like for , for
, for 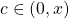 and for
and for 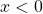 , the case is same because in either way
, the case is same because in either way 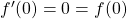
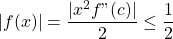
so when we apply
For problem 3, can we use the Taylor’s theorem instead? like for , for
, for 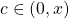 and for
and for 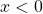 , the case is same because in either way
, the case is same because in either way 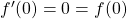
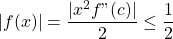
so when we apply
#1) similar sol. for question 3rd:
i.e. Maclaurin’s theorem can we used.
#2) and i think for question no. 10th: probability section i.e. in case of P[B] , there is a calculation mistake …
if not then i have doubt how { (1-p)^ 2n – k – l – 3 } comes?
because i concluded it is not -3 , it would be -4.
please correct me.
#3) for question no. 4th:
can we check limit along the curve y = x^2/3 which can also shows that f is not continuous?
#4) for |G| = pq
if we take a example of o(G) = 10 = 1*2*5
let p=2 < q=5 and both are prime.
and there is an element of order 1 (identity element) and it is non abelian group i.e. no element exist of order 10.
now phi(q) == phi(5) == (5^1-5^0) == 4
that is we can say only four elements are there of order q. And remaining elements could be of order p i.e. there are 4 elements of
order q.
Also q=5 implies that no. of elements of order q = q -1
And no. of elements of order p == o(G) – identity element – (q-1) == (p-1)q
can it be possible ?
if not give the query please.
sorry for #2)
it was my mistake.
For the 6th problem, It easily follows from the Sylow’s theorem.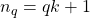 for some
for some  and
and 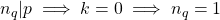 i.e there exist a unique subgroup of order
i.e there exist a unique subgroup of order 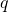 . so there are
. so there are 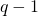 elements of order
elements of order 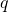
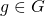 has order either
has order either 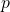 or
or 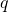 . So the remaining elements must have order
. So the remaining elements must have order 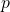
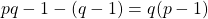
No. of q-sylow subgroup is,
and we know that every non-identity element in
Therefore the no. of elements having order q is
Sir,I am from Midnapore college ,W.B and I have decided to appear in ISI.math entrance.But I have no guide.So,I am quite confused about what type of preparation and study is needed.Please suggest me something in this regard.
I have already written everything you need to know on how to prepare for the ISI M.Math entrance exam here: https://fractionshub.com/courses/i-s-i-m-math-i-i-t-jam-exam-preparation/
Hello SIr, I am preparing for ISI MMath Entrance. Can you please provide my some training for problem solving. Is there any mock test series for ISI MMath
You will have to join the MMath group here. I teach for MMath entrance exam. No there is no mock tests for ISI MMath.
Great work…
Can you provide your what’s app group?
You can text me on WhatsApp @ 9700803692.
For problem 9, Here’s an alternative solution: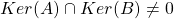 then we are done. Otherwise assume that
then we are done. Otherwise assume that 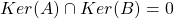 , also note that
, also note that  then
then  . So we have
. So we have  as dim(Ker (A) \cap ker(B)) =0 but from the given condition we have
as dim(Ker (A) \cap ker(B)) =0 but from the given condition we have 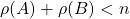 we have
we have 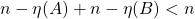 i.e
i.e  but from the above inequality we get a contradiction. Hence
but from the above inequality we get a contradiction. Hence 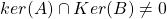 . Therefore the result follows
. Therefore the result follows
if
For problem 9, Here’s an alternative solution: then we are done. Otherwise assume that
then we are done. Otherwise assume that  , also note that
, also note that  then
then  . So we have
. So we have as
as 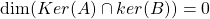 but from the given condition we have
but from the given condition we have 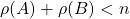 we have
we have 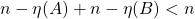 i.e
i.e  but from the above inequality we get a contradiction. Hence
but from the above inequality we get a contradiction. Hence 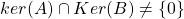 . Therefore the result follows
. Therefore the result follows
if
Sir ,your solution is really very helpful..
But if you provide us crash course for preparing M math ..
It will be very helpful…
For problem 2 can we use sandwich theorem instead,
Since g : [0,1] –> R, it is a continuous function from a compact set, thus its image will be compact.
|g(x)| 0
Let k be the supremum
for x belongs to [0,1)
-M(x^n) <= (x^n) g(x) <= k <= M (x^n)
applying limit and sandwich theorem, we get k tends to 0, and for x=1 since g(1)=0, thus (1^n)(g(1))=0, thus the limit always tends to zero.